Half life
Half life is the time that it takes for half of the original value of some amount of a radioactive element to decay. This also implies that one half life is the time that it takes for the activity of a source to fall to half its original value.[2] These radioactive atoms release energy to become new, different types of atoms at some measurable rate known as radioactive decay.
All radioactive materials have unstable nuclei within them; these are nuclei that will decay. In addition, there are also some nuclei within the substance that are already in their stable state but the proportion of stable to unstable nuclei in a sample can vary. The stable nuclei in the sample are unchanging (and in a stable energetic state), but the unstable nuclei will undergo some sort of nuclear decay over time to become stable.[3] This results in an emission of some form of energy that travels along a 'ray', which is why we call it radiation. Since half life is a measure of time, the half life is a value that determines how long this reduction to a more stable energy state will take.[2]
Different substances experience a loss of their radioactivity more quickly than others. Some radioactive elements have half of their unstable nuclei decay in much less than one second. For example, krypton-101 has a half life of about a ten millionth of a second.[4] In contrast, some elements have extraordinarily long half lives and take billions of years to decay. Uranium-238 has a half life of 4.51 billion years.[2] This means that it would take billions of years for uranium-238 to decay into a ratio of half uranium-238 and half thorium-234.[4] Uranium-235 (another naturally occurring isotope of uranium) has a shorter half life than uranium-238, that's only ~700 million years.[4]
Equation
There is an equation that is frequently used to determine how much of a certain radioactive substance remains after a given time has passed. This is determined from properties such as the half life of the substance, and how much of the substance there was initially. The equation used is:
where:
- is the amount of substance after time has passed
- is the initial amount of substance
- is the amount of time that has passed
- is the half life of the substance
A similar equation can be used to show how the activity of the substance diminishes over time. When this is being expressed, the equation takes the form:
where:
- is the activity of the substance after time has passed
- is the initial activity of the substance
- is the amount of time that has passed
- is the half life of the substance
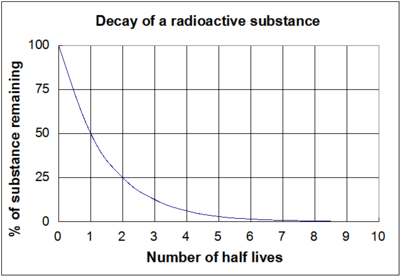
The graph shown in Figure 1 is a visual representation of these equations above. It is important to note that regardless of the actual length of the half-life (whether it is millions of years or a few nanoseconds) the shape of the graph will be the same.
Knowledge of half lives is part of how geologists date rocks with radioisotopic dating.
For Further Reading
- Radioactive
- Radioactive vs irradiated
- Radioactive decay
- Second law of thermodynamics
- Or explore a random page
References
- ↑ Created internally by a member of the Energy Education team
- ↑ 2.0 2.1 2.2 GCSE Physics. (July 23, 2015). Half Life [Online]. Available: http://www.gcsescience.com/prad16-half-life.htm
- ↑ HyperPhysics. (July 23, 2015). Radioactive Half-Life [Online]. Available; http://hyperphysics.phy-astr.gsu.edu/hbase/nuclear/halfli.html
- ↑ 4.0 4.1 4.2 Chart of the nuclides. (July 24, 2015). Half-Life [Online]. Available; http://www.nndc.bnl.gov/chart/reCenter.jsp?z=92&n=143

