Isothermal
Isothermal refers to a process in which a system changes—whether it be the pressure, volume and/or contents—without the temperature changing. From the point of view of the first law of thermodynamics, this means that the internal energy of the system is unchanged, since temperature is a measure of the average kinetic energy of molecules within the system.[1] The total energy of the system (and the enthalpy) often changes in isothermal processes though. The first law of thermodynamics can be expressed mathematically as:
Which can be simplified to show that the amount of heat and work is exactly equal when there's no change in temperature:
where:
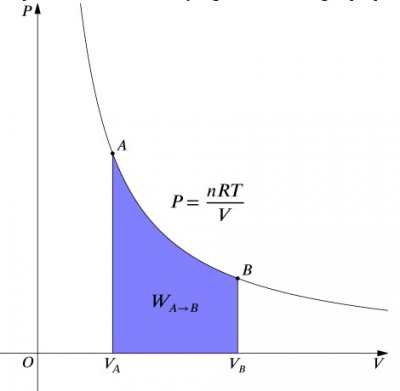
This expression means that the work input to a system must be exactly balanced by a heat output, and vice versa. If an insulated container containing air is compressed (decreasing its volume, positive value), then heat must be removed from the system in accordance (negative value). In contrast, if a container is allowed to expand (negative ), then heat must be added to the system in order to keep the temperature constant. To calculate work, an integration must be done to the formula Failed to parse (syntax error): {\displaystyle W = -∫pdV} . This can also be thought of as calculating the area under the curve. However, due to the shape of the curve, it's not as simple of a calculation—in comparison to an isobaric process, for example. The formula below is the integrated equation, and will calculate the work done for any isothermal process:
where:
- is the number of moles
- is the ideal gas constant
- is the initial pressure
- is the final volume
The Carnot efficiency explaining the maximum thermal efficiency of a heat engine is derived by using isothermal processes, in which a thermodynamic cycle is completed with the use of 2 isothermal and 2 adiabatic processes.[3] Phase changes are an example of isothermal processes since the temperature remains constant until the phase change is complete.
The following video from UC Berkley's chemistry department explains the idea of an isothermal process with visuals.
Full Integration of the Work Equation
References
- ↑ H. Gould and J. Tobochnik, "Temperature," in Statistical and Thermal Physics, 1st ed., Princeton, NJ: Princeton University Press, 2010, ch.2, sec.4, pp. 35-38
- ↑ R. Knight, Physics for scientists and engineers. San Fransisco: Pearson Addison Wesley, 2008, p. 512.
- ↑ R. D. Knight, "The Limits of Efficiency" in Physics for Scientists and Engineers: A Strategic Approach, 3nd ed. San Francisco, U.S.A.: Pearson Addison-Wesley, 2008, ch.19, sec.5, pp. 540-542

